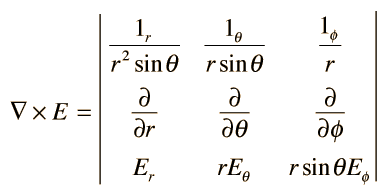The Curl

The curl of a vector function is the vector product of the del operator with a vector function:
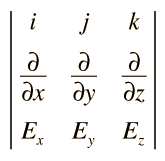
where i,j,k are unit vectors in the x, y, z directions. It can also be expressed in determinant form:

Curl in cylindrical and sphericalcoordinate systems

Applications:London equation for superconductors
 Index

Vector calculus
 
HyperPhysics*****HyperMath*****CalculusR Nave
 Go Back





Curl, Cylindrical

The curl in cylindrical polar coordinates, expressed in determinant form is:

 Index

Vector calculus
 
HyperPhysics*****HyperMath*****CalculusR Nave
 Go Back





Curl, Spherical

The curl in spherical polar coordinates, expressed in determinant form is:

 Index

Vector calculus
 
HyperPhysics*****HyperMath*****CalculusR Nave
 Go Back