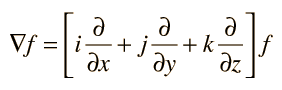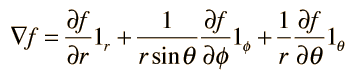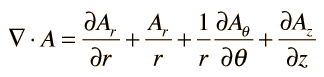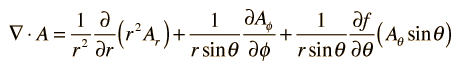The Gradient
The gradient is a vector operation which operates on a scalar function to produce a vector whose magnitude is the maximum rate of change of the function at the point of the gradient and which is pointed in the direction of that maximum rate of change. In rectangular coordinates the gradient of function f(x,y,z) is:
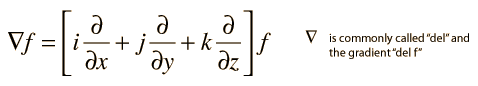
If S is a surface of constant value for the function f(x,y,z) then the gradient on the surface defines a vector which is normal to the surface.
| Applications | Conduction heat transfer |
The divergence of the gradient is called the LaPlacian. It is widely used in physics.
Gradient in other coordinate systems
Vector calculus
| HyperPhysics*****HyperMath*****Calculus | R Nave |