Just Temperament

Just temperament refers to a musical scale or musical intervals which maintain exact integer ratios between pitches. For example, the ration 3:2 is said to be a "just" musical fifth and is sometimes called a "perfect fifth". Pythagorean temperament maintains just intervals for the fifth and fourth but departs for some other intervals. Equal temperament does not contain any just intervals except the octave itself. However, if five cents is taken as the just noticeable difference in pitch, the fifths and fourths of equal temperament are just within that 5¢ margin.

Quarter-comma Meantone Tuning

Pentatonic Scale

A five-note pentatonic scale can be built up with a circle of fifths, a strategy based on our understanding of the human hearing response to small integer ratios of frequencies.

The Pythagorean scale can be produced by carving a natural whole tone out of the larger interval which naturally appears in the pentatonic scale.

The Pythagorean scale formed by the addition of the notes E and B to the pentatonic scale establishes the pattern for current western musical scales. That pattern is indicated by

where W represents a whole tone and H a semitone, and such a scale is referred to as a "diatonic" scale.

Diatonic and Chromatic Scales

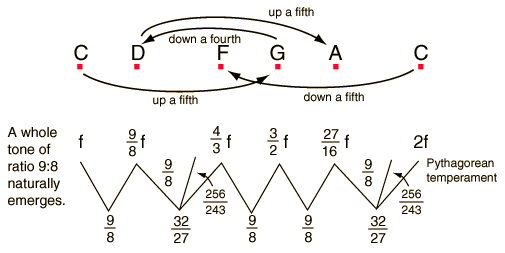
Building up a musical scale using a sequence or cycle of musical fifths and fourths leads first to the pentatonic scale, but this leaves two large intervals which in the illustration below would be labeled D-F and A-C. If you take a whole tone interval out of one of the large intervals, a smaller interval or semitone remains. In this way it could be said that the semitone inevitably arises from the pattern of buildup by fifths and fourths. The pattern which results in our example of the Pythagorean scale is the sequence WWHWWWH.

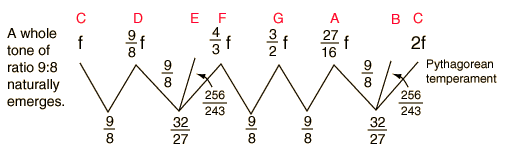
A scale with this sequence is called a diatonic scale. With our choice of C as the starting point, this example has no sharps or flats. When the whole tones of this diatonic scale are divided into semitones with additional notes, these are called chromatic notes and the scale where they are included is called a chromatic scale. In this particular example, all the chromatic notes added would be denoted by sharps or flats. An entire chromatic scale in current musical keys would consist of octaves with 12 semitones or half-steps.

Note that it is not the absence of sharps and flats which defines the diatonic scale - that would depend upon the starting point once you have associated letter names ABC... with the notes. The defining criterion for the diatonic scale is the sequence of whole and half-steps, WWHWWWH.

On the piano keyboard, an example of the equal tempered scale, the pattern WWHWWWH is demonstrated by the pattern of the white keys if you start with middle-C as indicated. The white keys that have a black key between them are a whole tone apart, but the E-F and B-C white keys do not have a black key between and are a semitone apart.