Ferromagnetism

Iron, nickel, cobalt and some of the rare earths (gadolinium, dysprosium) exhibit a unique magnetic behavior which is called ferromagnetism because iron (ferrum in Latin) is the most common and most dramatic example. Samarium and neodymium in alloys with cobalt have been used to fabricate very strong rare-earth magnets.

Ferromagnetic materials exhibit a long-range ordering phenomenon at the atomic level which causes the unpaired electron spins to line up parallel with each other in a region called a domain. Within the domain, the magnetic field is intense, but in a bulk sample the material will usually be unmagnetized because the many domains will themselves be randomly oriented with respect to one another. Ferromagnetism manifests itself in the fact that a small externally imposed magnetic field, say from a solenoid, can cause the magnetic domains to line up with each other and the material is said to be magnetized. The driving magnetic field will then be increased by a large factor which is usually expressed as a relative permeability for the material. There are many practical applications of ferromagnetic materials, such as the electromagnet.

Ferromagnets will tend to stay magnetized to some extent after being subjected to an external magnetic field. This tendency to "remember their magnetic history" is called hysteresis. The fraction of the saturation magnetization which is retained when the driving field is removed is called the remanence of the material, and is an important factor in permanent magnets.

All ferromagnets have a maximum temperature where the ferromagnetic property disappears as a result of thermal agitation. This temperature is called the Curie temperature.

Ferromagntic materials will respond mechanically to an impressed magnetic field, changing length slightly in the direction of the applied field. This property, called magnetostriction, leads to the familiar hum of transformers as they respond mechanically to 60 Hz AC voltages.

Long Range Order in Ferromagnets

The long range order which creates magnetic domains in ferromagnetic materials arises from a quantum mechanical interaction at the atomic level. This interaction is remarkable in that it locks the magnetic moments of neighboring atoms into a rigid parallel order over a large number of atoms in spite of the thermal agitation which tends to randomize any atomic-level order. Sizes of domains range from a 0.1 mm to a few mm. When an external magnetic field is applied, the domains already aligned in the direction of this field grow at the expense of their neighbors. If all the spins were aligned in a piece of iron, the field would be about 2.1 Tesla. A magnetic field of about 1 T can be produced in annealed iron with an external field of about 0.0002 T, a multiplication of the external field by a factor of 5000! For a given ferromagnetic material the long range order abruptly disappears at a certain temperature which is called the Curie temperature for the material. The Curie temperature of iron is about 1043 K.

The Curie Temperature

For a given ferromagnetic material the long range order abruptly disappears at a certain temperature which is called the Curie temperature for the material. The Curie temperature of iron is about 1043 K. The Curie temperature gives an idea of the amount of energy takes to break up the long-range ordering in the material. At 1043 K the thermal energy is about 0.135 eV compared to about 0.04 eV at room temperature.

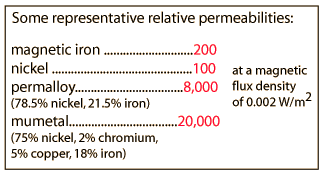
Magnetic Domains

The microscopic ordering of electron spins characteristic of ferromagnetic materials leads to the formation of regions of magnetic alignment called domains.

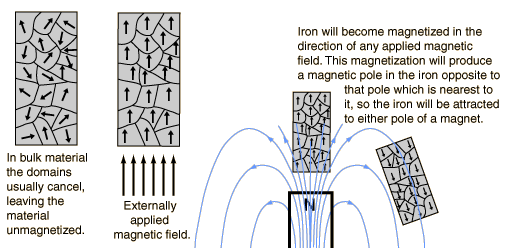
The main implication of the domains is that there is already a high degree of magnetization in ferromagnetic materials within individual domains, but that in the absence of external magnetic fields those domains are randomly oriented. A modest applied magnetic field can cause a larger degree of alignment of the magnetic moments with the external field, giving a large multiplication of the applied field.

These illustrations of domains are conceptual only and not meant to give an accurate scale of the size or shape of domains. The microscopic evidence about magnetization indicates that the net magnetization of ferromagnetic materials in response to an external magnetic field may actually occur more by the growth of the domains parallel to the applied field at the expense of other domains rather than the reorientation of the domains themselves as implied in the sketch.

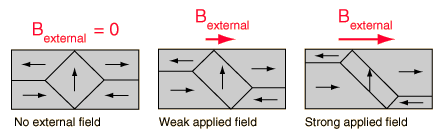
Some of the more direct evidence we have about domains comes from imaging of domains in single crystals of ferromagnetic materials. The sketches above are after Young and are adapted from magnified images of domain boundaries in single crystals of nickel. They suggest that the effect of external magnetic fields is to cause the domain boundaries to shift in favor of those domains which are parallel to the applied field. It is not clear how this applies to bulk magnetic materials which are polycrystalline. Keep in mind the fact that the internal magnetic fields which come from the long range ordering of the electron spins are much stronger, sometimes hundreds of times stronger, than the external magnetic fields required to produce these changes in domain alignment. The effective multiplication of the external field which can be achieved by the alignment of the domains is often expressed in terms of the relative permeability.

Domains may be made visible with the use of magnetic colloidal suspensions which concentrate along the domain boundaries. The domain boundaries can be imaged by polarized light, and also with the use of electron diffraction. Observation of domain boundary movement under the influence of applied magnetic fields has aided in the development of theoretical treatments. It has been demonstrated that the formation of domains minimizes the magnetic contribution to the free energy.

Relative Permeability

The magnetic constant μ₀ = 4π x 10^-7 T m/A is called the permeability of space. The permeabilities of most materials are very close to μ₀ since most materials will be classified as either paramagnetic or diamagnetic. But in ferromagnetic materials the permeability may be very large and it is convenient to characterize the materials by a relative permeability.

Table of magnetic properties

When ferromagnetic materials are used in applications like an iron-core solenoid, the relative permeability gives you an idea of the kind of multiplication of the applied magnetic field that can be achieved by having the ferromagnetic core present. So for an ordinary iron core you might expect a magnification of about 200 compared to the magnetic field produced by the solenoid current with just an air core. This statement has exceptions and limits, since you do reach a saturation magnetization of the iron core quickly, as illustrated in the discussion of hysteresis.

Applications of Ferromagnetism