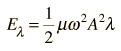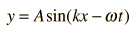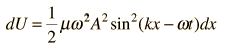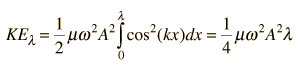How much power is transported by a string wave?

As a sinusoidal wave moves down a string, the energy associated with one wavelength on the string is transported down the string at the propagation velocity v. From the basic wave relationship, the distance traveled in one period is vT = λ, so the energy is transported one wavelength per period of the oscillation.

The energy associated with one wavelength of the wave is

Show

so the transported power is

Energy in a String Wave

The energy associated with a traveling wave in a stretched string is conveniently expressed as the energy per wavelength.

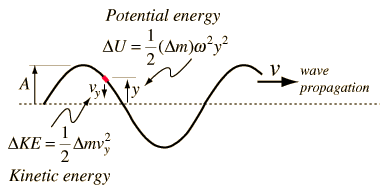
The energy of a small segment of the string can be expressed as the sum of the kinetic energy and elastic potential energy of the segment. The differential form of the elastic potential energy is

Using the description of a traveling wave

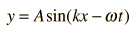
the potential energy expression becomes

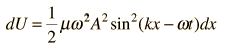
The energy for a full wavelength can be found by integrating this expression at a given time, and it is most convenient to set t=0 for this integration. The energy for one wavelength along the string is

The differential kinetic energy is

Using the velocity expression

the kinetic energy takes the form

and again setting t=0 for simplification

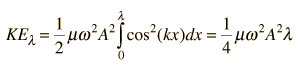
The total energy associated with a wavelength is

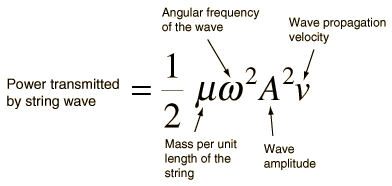
Since this amount of energy is transported a distance of one wavelength along the string in one period, this expression can be used to calculate the power transmitted along a string.