Quantized Energy States

Angular Momentum Quantization

and the standing wave condition that circumference = whole number of wavelengths. In the hydrogenic case, the number n is the principal quantum number.

These can be combined to get an expression for the angular momentum of the electron in orbit. (Note that this assumes a circular orbit, a generally unwarranted assumption.)

Use in Bohr orbit

Classical Electron Orbit

In t he Bohr theory, this classical result was combined with the quantization of angular momentum to get an expression for quantized energy levels.

Bohr Orbit

Wavelengths for Different States

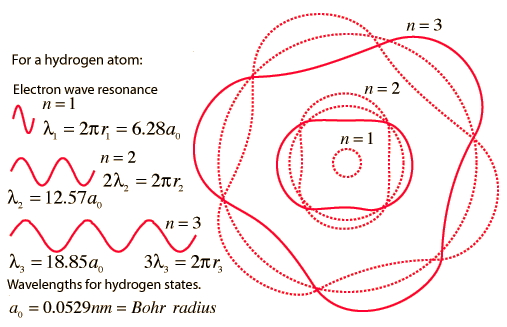
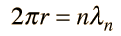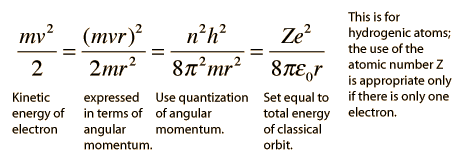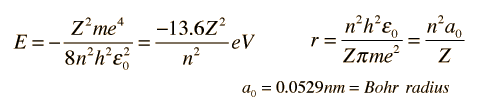Failures of the Bohr Model

While the Bohr model was a major step toward understanding the quantum theory of the atom, it is not in fact a correct description of the nature of electron orbits. Some of the shortcomings of the model are:

1. It fails to provide any understanding of why certain spectral lines are brighter than others. There is no mechanism for the calculation of transition probabilities.

2. The Bohr model treats the electron as if it were a miniature planet, with definite radius and momentum. This is in direct violation of the uncertainty principle which dictates that position and momentum cannot be simultaneously determined.

The Bohr model gives us a basic conceptual model of electrons orbits and energies. The precise details of spectra and charge distribution must be left to quantum mechanical calculations, as with the Schrodinger equation.